Structured Markov Chains Arising from Finite-Source Retrial Queues with Orital Search

Abstract

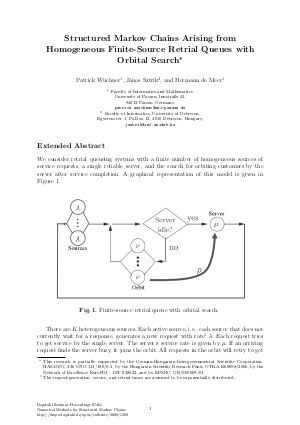
We consider retrial queueing systems with a finite number of 
homogeneous sources of calls, a single reliable server, and the 
search for orbiting customers by the server after job completion. 
During this investigation, the infinitesimal generator of the 
underlying (finite) continuous-time Markov chain takes a 
(level-dependent) QBD-like form.

After solving for the steady state probabilities using the MOSEL-2 
tool, the results show a surprising maximum of the mean response 
time. This maximum was already discovered by other researchers 
dealing with finite-source retrial queues. However, to our best 
knowledge, no thorough investigation was done yet why this 
maximum exists and in which way it depends on the system 
parameters.

In the talk, after introducing the backgrounds of finite-source 
retrial queues with orbital search, a generalized stochastic 
Petri net is used to derive the underlying continuous-time 
Markov chain and its generator. Finally, using the seminar, 
we can hopefully bring forward discussions how to make more
general statements on the parameter-dependent behavior of 
the response time’s maximum.