Local Limit of Random Discrete Surface with (Or Without!) a Statistical Physics Model (Invited Talk)

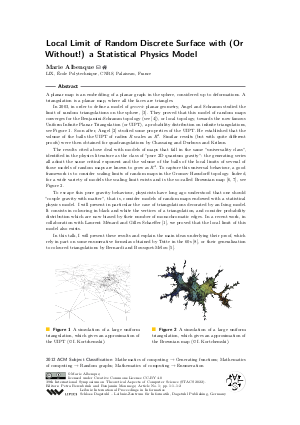
A planar map is an embedding of a planar graph in the sphere, considered up to deformations. A triangulation is a planar map, where all the faces are triangles. In 2003, in order to define a model of generic planar geometry, Angel and Schramm studied the limit of random triangulations on the sphere, [Angel and Schramm, 2003]. They proved that this model of random maps converges for the Benjamini-Schramm topology (see [Benjamini and Schramm, 2001]), or local topology, towards the now famous Uniform Infinite Planar Triangulation (or UIPT), a probability distribution on infinite triangulations, see Figure 1. Soon after, Angel [Angel, 2003] studied some properties of the UIPT. He established that the volume of the balls the UIPT of radius R scales as R⁴. Similar results (but with quite different proofs) were then obtained for quadrangulations by Chassaing and Durhuus and Krikun. The results cited above deal with models of maps that fall in the same "universality class", identified in the physics literature as the class of "pure 2D quantum gravity": the generating series all admit the same critical exponent and the volume of the balls of the local limits of several of those models of random maps are known to grow as R⁴. To capture this universal behaviour, a good framework is to consider scaling limits of random maps in the Gromov Hausdorff topology. Indeed, for a wide variety of models the scaling limit exists and is the so-called Brownian map [Le Gall, 2013; Miermont, 2013], see Figure 2. To escape this pure gravity behaviour, physicists have long ago understood that one should "couple gravity with matter", that is, consider models of random maps endowed with a statistical physics model. I will present in particular the case of triangulations decorated by an Ising model. It consists in colouring in black and white the vertices of a triangulation, and consider probability distribution which are now biased by their number of monochromatic edges. In a recent work, in collaboration with Laurent Ménard and Gilles Schaeffer [Albenque et al., 2020], we proved that the local limit of this model also exists. In this talk, I will present these results and explain the main ideas underlying their proof, which rely in part on some enumerative formulas obtained by Tutte in the 60s [Tutte, 1962], or their generalization to coloured triangulations by Bernardi and Bousquet-Mélou [Bernardi and Bousquet-Mélou, 2011].